11 Basic Classical Geometries in
Architecture
11 Classical Architectural Geometries
The type and use of geometry in architecture is a fundamental element of the variation between styles or language of architecture. The type of geometry is often conflated with meaning. One concept is that architectural style-isms like art swing between periods of “romanticism” and “realism”. With “romantic” being soft, using decoration and curvature, and “realism” being stripped down and hard edged. Another concept is that the swing is between the metaphysical/godly and the worldly/functional. Any such simplification is just that, a simplification. And this needs to be kept in mind when reading the following.
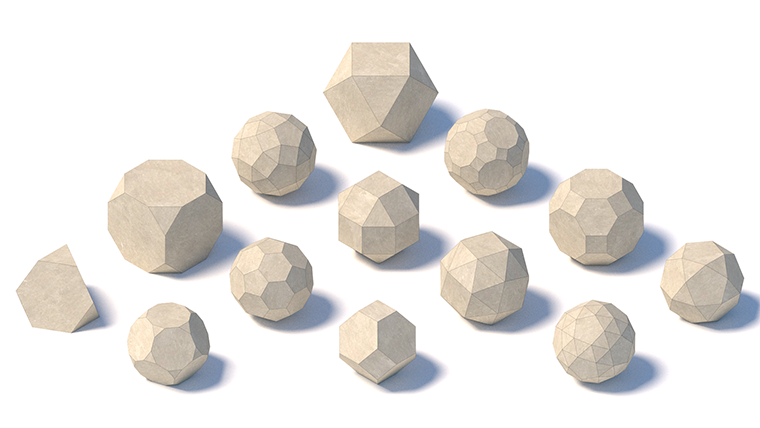
The 5 Platonic solids:
Named for Plato who in the Timaeus dialogues, hypothesized that the classical elements of earth, water air and fire were made of these regular solids.
Platonic solid is a regular, convex polyhedron. It is constructed by matching side, regular, polygonal faces. With the same number of faces meeting at each corner. The five solids are Cube, Tetrahedron, Dodecahedron, Icosahedron, and Octahedron.
The 13 Archemedian solids:
Named after Archemedes who discussed them in a now lost work. Unlike the Platonic solids the Archemedian solids are not identical, but convex uniform polyhedra composed of regular polygons meeting in identical vertices. The 13 solids are:
- Truncated Tetrahedron
- Truncated Cube
- Rhombicosidodecahedron (Small Rhombicosidodecahedron)
- Cuboctahedron (Rhombitetratetrahedron)
- Truncated Dodecahedron
- Truncated Icosahedron
- Rhombicuboctahedron (Small Rhombicuboctahedron)
- Truncated Icosidodecahedron (Great Rhombicosidodecahedron)
- Truncated Octahedron (Truncated Tetratetrahedron)
- Snub Cube (Snub Cuboctahedron)
- Truncated Cuboctahedron (Great Rhombicuboctahedron)
- Snub Dodecahedron (Snub Icosidodecahedron)
- Icosidodecahedron
Golden Section
The golden ratio is where two quantities have a ratio that is the same as the ratio of their sum to the larger of the two quantities. If you cut a square from a golden ratio rectangle, you are left with a smaller golden ratio rectangle. The ratio is app. 1 to 1.618. This ratio is considered to have a mystical and aesthetic quality used from ancient Greek architecture to Modernists like Le Corbusier.
Fibonacci numbers
Leading directly from the golden ratio Fibonacci numbers are closely related. The sequence is where each number is the sum of the previous two numbers: 0, 1, 1, 2, 3, 5, 8, 13, 21, 34 etc. This ratio is the same as the sequential reduction of divided golden section (square) sides. This combination leads to the golden spiral.
Projected Geometry
In order to scale, one has to be able to project one geometry to another. Classically this would be done by hand to eye coordination. Later scaled down models and drawings would work as a representation/instruction of the proposed work. This scaling would have implication on the geometry and language of the architecture.
Piling
A structural support construction material heaped on top of one another. Classical approach includes piling different types of materials to form banded patterns, or carving decorations into stacked stone structures.
Tessellation
Classically a flat surface covered in tiles forming a geometric pattern. The tiling can include regular or irregular patterns, periodic or non-periodic. Tessellation is found in nature, such as honeycomb patterns.
Periodic tiling
Surface applied covering made of multiple tiles. Periodic tiling on a 2D surface will repeat in both directions
Aperiodic tiling (Penrose tiling)
Non-periodic tiling where the pattern does not contain arbitrary large periodic patches. Penrose tiling in the best known (and interesting) example of this type of tiling.
Origami
Originally an art of paper folding, the classical geometries method is closely related to tessellation using a single surface material. Origami spans the area of classical to modern/contemporary geometry in architecture.
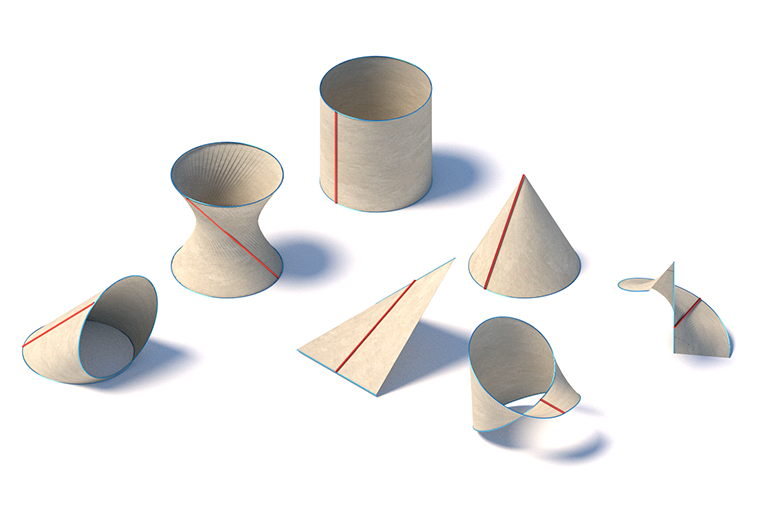
Ruled Surfaces
A surface is a set of points swept by a moving straight line. In essence a 3 dimensional surface that can be created with straight lines, allowing the forming of organic looking surfaces using simple tools. Ruled surfaces, such as hyperboloid structures have been made throughout history. Antonio Gaudi used ruled surfaces as the base to develop the complexities of his architecture. Ruled surfaces include Cylinders, Cones, Helicoids, Hyperboloids, Hyperbolic Parabloids and Möbius strips.
Gothic
Gothic architecture (from the Normans to Gaudi) is based on synthesis and direction of forces that are both used structurally and made visible. The process generates ideal forms based on structure over planned space. While classical architecture attempted to apply the properties of a given materials to a form, Gothic architecture used the properties of the material to give the form.
Combination
The combination and variations of classical approaches to geometry go right they way into classical modernism. The more traditional practitioners lament the disappearance of classical geometries from architecture and blame this for a fall in aesthetic understanding amongst the profession and general public. On the other side are contemporary architects like Patrick Schumacher calling the approach repetition of primitives, like a garbage heap.
Readers will have to make their own choice where they fall on the matter, but as with all evolutions of styles and methods, the new is usually never exclusive from the old.
Next, contemporary geometries…
By Gudjon Thor Erlendsson
© 2020 Gudjon Thor Erlendsson, all rights reserved.